Variation of Pressure in Liquids with depth
Variation of Pressure in Liquids with depth
Variation of Pressure in Liquids With Depth: When we swim in a river, we feel the water pressure acting against our eardrums. The deeper we swim, the greater the pressure. The cause of this pressure is the weight of the fluid, water plus air directly above us. As we swim deeper, there is more water above us. Thereofore, there is more pressure. If we swim twice as deep, there is twice the weight of water above us, so the water contribution to the pressure felt by us is doubled. The pressure due to a liquid also depends upon its density.
Mathematical expression for fluid pressure
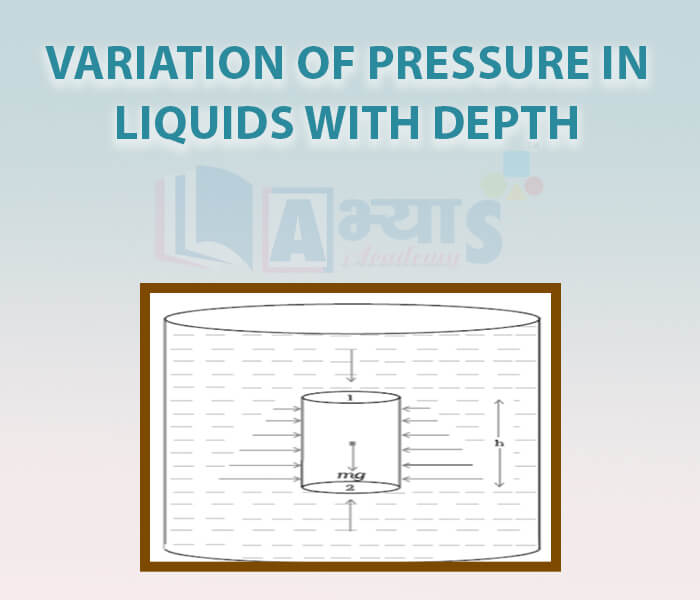
Let us consider a liquid of density in a beaker of base area A. If h be the height of the liquid column then
Volume of liquid in beaker,
Force (or thrust) acting at the bottom of the beaker is given by
F = Weight of liquid = mg
Pressure at the bottom of the beaker
Thus, we observe that pressure at a depth h due to a liquid column is directly proportional to
Q. Calculate the average density of the atmosphere, given that it extends to an altitude of 120 km. Compare this density with that of the air.
Solution : If we solve
Here, we can take P = atmospheric pressure, h (height) is known and g(accelaeration due to garvity) also known.
Entering the value in the value of ,
Q. Calculate the depth below the surface of water at which the pressure due to the weight of water equals 1.00 atm.
Solution: As we know that
The depth of liquid increases, the weight of liquid column pushing down from above increases, and the pressure _________________________. | |||
| Right Option : A | |||
| View Explanation | |||
The greater the height, the _______________________ the pressure. | |||
| Right Option : A | |||
| View Explanation | |||
Which of the following are correct : (a) The deeper we swim, the greater the pressure. (b) The deeper we swim, the smaller the pressure. (c) The pressure due to a liquid depends upon its density. | |||
| Right Option : C | |||
| View Explanation | |||
Students / Parents Reviews [20]
About Abhyas metholodology the teachers are very nice and hardworking toward students.The Centre Head Mrs Anu Sethi is also a brilliant teacher.Abhyas has taught me how to overcome problems and has always taken my doubts and suppoeted me.
Shreya Shrivastava
8thMy experience with Abhyas is very good. I have learnt many things here like vedic maths and reasoning also. Teachers here first take our doubts and then there are assignments to verify our weak points.
Shivam Rana
7thWhen I have not joined Abhyas Academy, my skills of solving maths problems were not clear. But, after joining it, my skills have been developed and my concepts of science and SST are very well. I also came to know about other subjects such as vedic maths and reasoning.
Sharandeep Singh
7thAbhyas is a complete education Institute. Here extreme care is taken by teacher with the help of regular exam. Extra classes also conducted by the institute, if the student is weak.
Om Umang
10thThe experience was nice. I studied here for three years and saw a tremendous change in myself. I started liking subjects like English and SST which earlier I ran from. Extra knowledge gave me confidence to overcome competitive exams. One of the best institutes for secondary education.
Aman Kumar Shrivastava
10thAbhyas is an institute of high repute. Yogansh has taken admission last year. It creates abilities in child to prepare for competitive exams. Students are motivated by living prizes on basis of performance in Abhyas exams. He is satisfied with institute.
Yogansh Nyasi
7thAbhyas academy is great place to learn. I have learnt a lot here they have finished my fear of not answering.It has created a habit of self studying in me.The teachers here are very supportive and helpful. Earlier my maths and science was good but now it has been much better than before.
Barkha Arora
10thIt was a good experience with Abhyas Academy. I even faced problems in starting but slowly and steadily overcomed. Especially reasoning classes helped me a lot.
Cheshta
10thIt was good as the experience because as we had come here we had been improved in a such envirnment created here.Extra is taught which is beneficial for future.
Eshan Arora
8thA marvelous experience with Abhyas. I am glad to share that my ward has achieved more than enough at the Ambala ABHYAS centre. Years have passed on and more and more he has gained. May the centre flourish and develop day by day by the grace of God.
Archit Segal
7thAbhyas is good institution and a innovative institute also. It is a good platform of beginners.Due to Abhyas,he has got knoweledge about reasoning and confidence.My son has improved his vocabulary because of Abhyas.Teacher have very friendly atmosphere also.
Manish Kumar
10thMy experience with Abhyas academy is very good. I did not think that my every subject coming here will be so strong. The main thing is that the online tests had made me learn here more things.
Hiya Gupta
8thUsually we see institutes offering objective based learning which usually causes a lag behind in subjective examinations which is the pattern followed by schools. I think it is really a work of planning to make us students grab the advantages of modes of examination, Objective Subjective and Onli...
Anika Saxena
8thBeing a parent, I saw my daughter improvement in her studies by seeing a good result in all day to day compititive exam TMO, NSO, IEO etc and as well as studies. I have got a fruitful result from my daughter.
Prisha Gupta
8thAbhyas institute is one of the best coaching institute in the vicinity of Ambala Cantt area. The teachers of the institute are well experienced and very helpful in solving the problems of the students.The good thing of the institute is that it is providing extra classes for the students who are w...
Aman Kumar Shrivastava
10thMy experience with Abhyas Academy has been very good. When I was not in Abhyas whenever teacher ask questions I could not speak it confidently but when I came in Abhyas, my speaking skills developed and now I am the first one to give the answer of teachers question.
Upmanyu Sharma
7thMy experience was very good with Abhyas academy. I am studying here from 6th class and I am satisfied by its results in my life. I improved a lot here ahead of school syllabus.
Ayan Ghosh
8thI have spent a wonderful time in Abhyas academy. It has made my reasoning more apt, English more stronger and Maths an interesting subject for me. It has given me a habbit of self studying
Yatharthi Sharma
10thIn terms of methodology I want to say that institute provides expert guidence and results oriented monitering supplements by requsite study material along with regular tests which help the students to improve their education skills.The techniques of providing education helps the students to asses...
Aman Kumar Shrivastava
10thMy experience with Abhyas academy is very nice or it can be said wonderful. I have been studying here from seven class. I have been completing my journey of three years. I am tinking that I should join Abhyas Academy in tenth class as I am seeing much improvement in Maths and English
